What is the Pythagorean Theorem?
The Pythagorean Theorem, also known as the theorem of the three squares, is a well-known theorem that describes a property of right-angled triangles. The content of this theorem is very simple: in a right-angled triangle, the sum of the squares of the two sides adjacent to the right angle is equal to the square of the length of the hypotenuse.
This can be expressed with the following formula:
a2 + b2 = c2
Here:
c is the hypotenuse (the side opposite the right angle and the longest side of the triangle).
a and b are the two sides that form the right angle, and
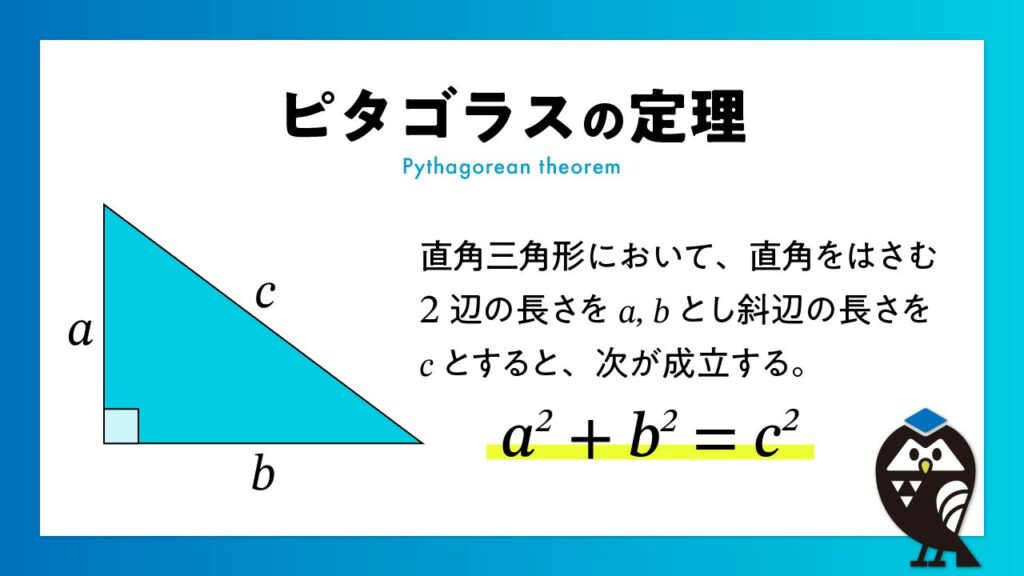
If the lengths of the three sides of a triangle, a, b, and c, satisfy the equation a² + b² = c², then the triangle is a right triangle with side c as the hypotenuse.
The Pythagorean Theorem is used to find the length of one side of a right triangle when the lengths of the other two sides are known. Let’s look at an example.
[Example Problem]
If one side of a right triangle is 3 cm and another side is 4 cm, what is the length of the hypotenuse?
[Solution]
Let the length of the hypotenuse be x, and apply the Pythagorean Theorem:
32 + 42 = x2
x2 = 9 + 16
x2 = 25
x = 5
The length of the hypotenuse is 5 cm.
As shown above, the Pythagorean Theorem makes it easy to calculate the length of a side.
Proof of the Pythagorean Theorem
It is said that there are over 100 different proofs of the Pythagorean Theorem. Here, we will introduce one of them.
Look at the figure below. Around a light blue square with side length c, draw four right triangles (blue) with base b and height a. This forms a larger square on the outside, with each side measuring a + b.
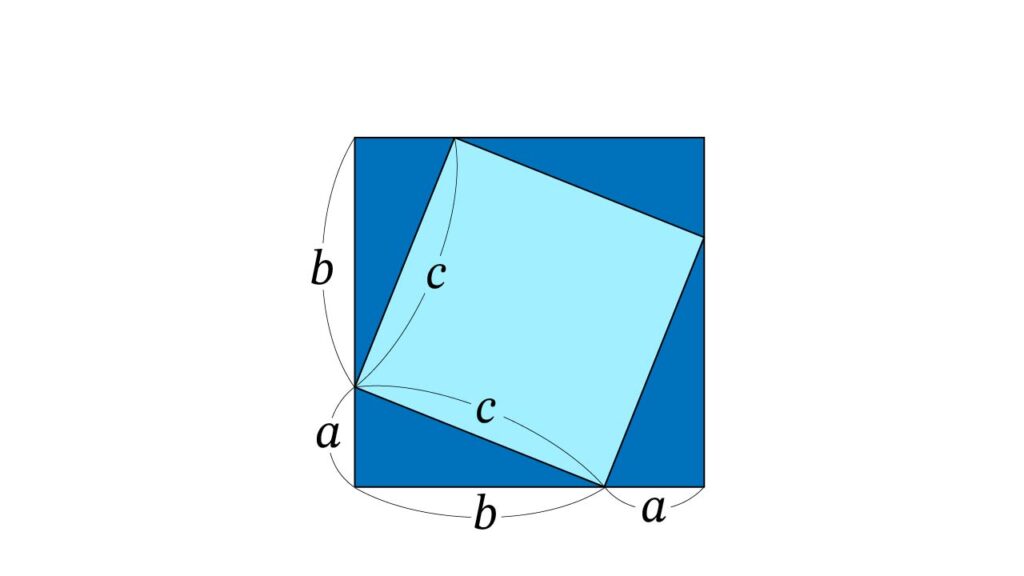
In this figure, the large outer square is composed of one square with side length c and four right triangles with base b and height a.
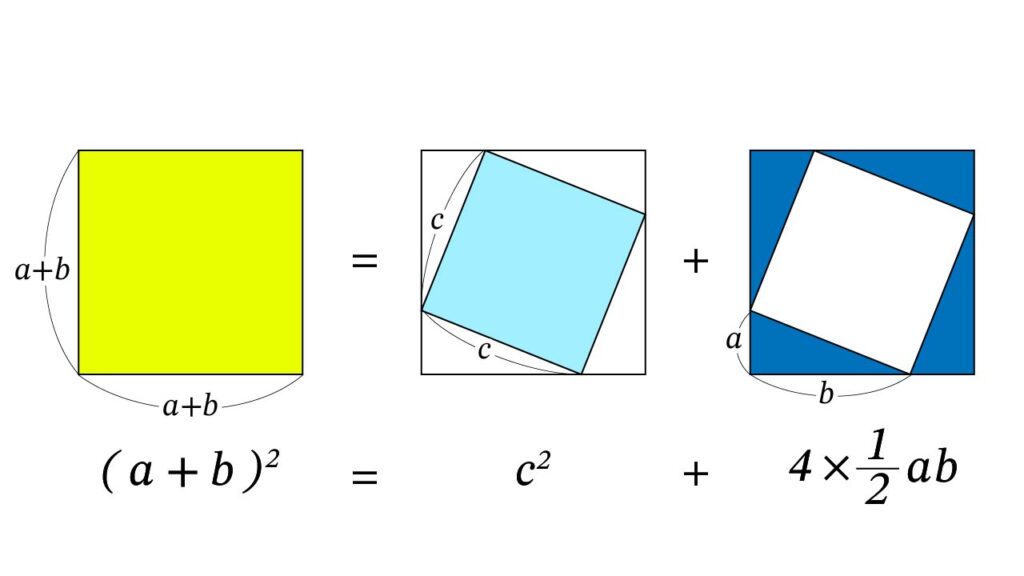
The area of the large outer square (in yellow) is (a + b)2, since each side measures a + b. This area is equal to the sum of the area of the inner square with side length c (in light blue) and the four right triangles with base b and height a (in blue). In equation form, this is expressed as follows:
( a+ b )2 = c2 + 4 × 1/2 ×ab
a2 + 2ab + b2 = c2 + 2ab
a2 + b2 = c2
Therefore, the Pythagorean theorem has been proven.
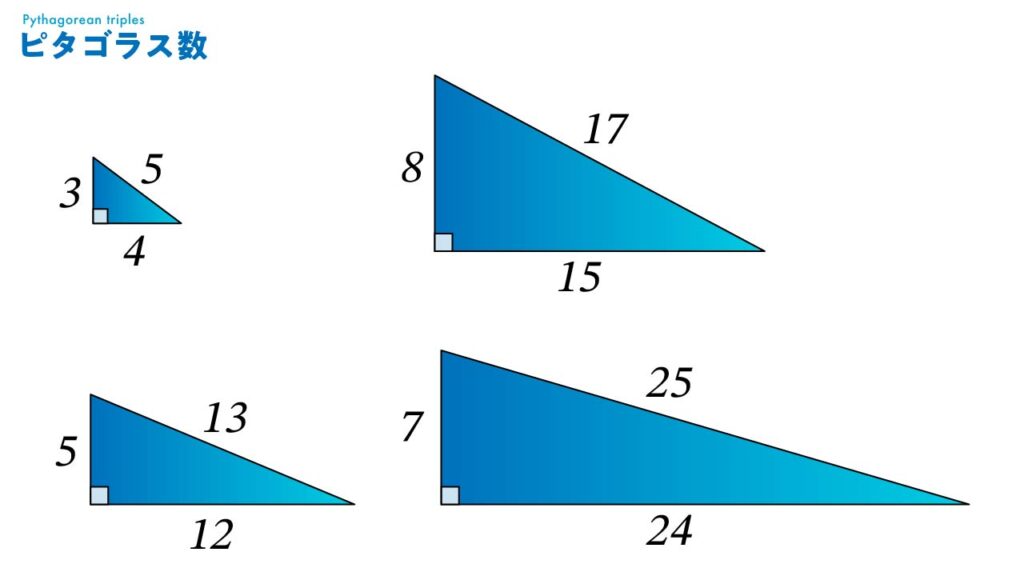
Pythagorean Triples
Three natural numbers a, b, c that satisfy the equation a2 + b2 = c2 are called Pythagorean numbers or Pythagorean triples. If (a, b, c) form a Pythagorean triple, then a triangle with sides of length a, b, and c will be a right triangle. The smallest and most well-known Pythagorean triple is (3, 4, 5), which some refer to as the original or classic Pythagorean triple.
The History of the Pythagorean Theorem
The properties of right triangles have been used since the time of ancient Egypt.
Egyptian civilization flourished along the Nile River. Every year, the Nile would flood at a predictable time, bringing fertile soil from upstream essential for growing crops. As the waters slowly receded, they left behind a muddy plain where the boundaries of farmland were no longer visible.
But there was no cause for concern—the land was managed by the state. Government officials known as “rope stretchers” conducted large-scale surveys, reorganized the agricultural land, and allocated plots to individual farmers.
To divide the land properly into farmland, it was essential to determine right angles. Using a single rope divided into lengths of 3, 4, and 5 units—with marks at the divisions—they could form a triangle. When stretched tight at the marked points, this triangle created a right angle.
In this way, the properties of right triangles were used in land surveying. While there is no direct evidence that the ancient Egyptians knew the full Pythagorean Theorem, it is clear they understood that the triple (3, 4, 5) would form a right triangle—that is, they knew that 32 + 42 = 52.